Spin spirals

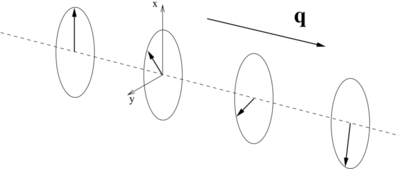
Generalized Bloch condition

Spin spirals may be conveniently modeled using a generalisation of the Bloch condition:

$\left[{\begin{array}{c}\Psi _{\bf {k}}^{\uparrow }({\bf {r)}}\\\Psi _{\bf {k}}^{\downarrow }({\bf {r)}}\end{array}}\right]=\left({\begin{array}{cc}e^{-i{\bf {q\cdot {\bf {R/2}}}}}&0\\0&e^{+i{\bf {q\cdot {\bf {R/2}}}}}\end{array}}\right)\left[{\begin{array}{c}\Psi _{\bf {k}}^{\uparrow }({\bf {r-R)}}\\\Psi _{\bf {k}}^{\downarrow }({\bf {r-R)}}\end{array}}\right]$

The above definition gives rise to the following magnetization density:

${\bf {m}}({\bf {r}}+{\bf {R}})=\left({\begin{array}{c}m_{x}({\bf {r}})\cos({\bf {q}}\cdot {\bf {R}})-m_{y}({\bf {r}})\sin({\bf {q}}\cdot {\bf {R}})\\m_{x}({\bf {r}})\sin({\bf {q}}\cdot {\bf {R}})+m_{y}({\bf {r}})\cos({\bf {q}}\cdot {\bf {R}})\\m_{z}({\bf {r}})\end{array}}\right)$